This uses the concepts of energy developed in Stage 1, Subtopic 4.1: Energy and Stage 2, Subtopic 2.2: Motion of charged particles in electric fields; momentum developed in Stage 1, Subtopic 4.2: Momentum; and waves developed in Stage 1, Subtopics 5.1: Wave model and 5.3 Light.
In interacting with matter, light behaves like particles (called ‘photons’), with energy given by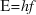 
and momentum given by 
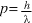where h is Planck’s constant, f is the frequency of the light, and 
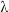is its wavelength.
- Solve problems using 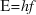and
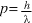
Electrons may be emitted from a metal surface when light of sufficiently high frequency is incident on the metal surface. This process is called the ‘photoelectric effect’.
If monochromatic light is used the intensity of the incident light affects the number, but not the energy, of emitted electrons.
The minimum frequency,
, at which electrons are emitted varies with the type of material and is called the ‘threshold frequency’.The work function, W, of a surface is the minimum energy required to remove an electron from it.
The work function is related to the threshold frequency by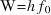 
- Describe an experimental method for investigating the relationship between the maximum kinetic energy of the emitted electrons, calculated from the measured stopping voltage using
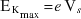and the frequency of the light incident on a metal surface. - Describe how Einstein used the concept of photons and the conservation of energy to explain the experimental observations of the photoelectric effect.
- Deduce the formula
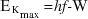where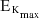 
is the maximum kinetic energy of the emitted electrons. - Plot experimental values of maximum kinetic energy vs frequency, and relate the slope and axes intercepts to the formula:
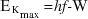
- Solve problems that require the use of
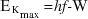
Photoelectric Effect Experiment Simulation
Video: The Photoelectric Effect
Particles exhibit wave behaviour with a wavelength (called the 'de Broglie wavelength') that depends on the momentum of the particle. The de Broglie wavelength is given by the formula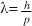 
where h is Planck’s constant and p is the momentum of the particles.
The wave behaviour of particles can be demonstrated using Young’s double-slit experiment and the Davisson–Germer experiment.
- Solve problems involving the use of the formula 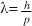for electrons and other particles.
- Describe two-slit interference pattern produced by electrons in double-slit experiments.
- Describe the Davisson–Germer experiment, in which the diffraction of electrons by the surface layers of a crystal lattice was observed.
- Compare the de Broglie wavelength of electrons with the wavelength required to produce the observations of the Davisson–Germer experiment and in two-slit interference experiments.
Davisson-Germer Experiment Simulation
Video: Double Slit Experiment (the last part about the observer is misleading though, ignore it)